Jim Belk Cornell University

Homework 6

Due Date: Friday, October 30

Let \(f\colon[0,8]\to[0,8]\) be the following piecewise-linear function.

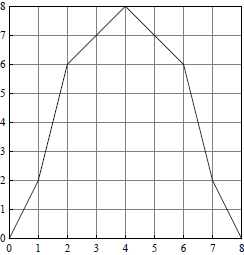\(\displaystyle f(x)\;=\;\begin{cases} 2x & \text{if }0 \leq x < 1, \\[6pt] 4x - 2 & \text{if }1 \leq x < 2, \\[6pt] x+4 & \text{if }2 \leq x < 4, \\[6pt] 12 - x & \text{if }4 \leq x < 6, \\[6pt] 30-4x & \text{if }6 \leq x < 7, \\[6pt] 16-2x & \text{if }7 \leq x \leq 8. \end{cases}\)

As with the tent map, we can make itineraries for \(f\), with \(\mathsf{L}=[0,4]\) and \(\mathsf{R}=[4,8]\). The goal of this assignment is to show that \(f\) is topologically conjugate to the tent map.

    1. The function \(f\) has one fixed point on the interval \((0,8)\). Find it.
    2. Find the point whose itinerary under \(f\) is \(\mathsf{LRRRRRR}\cdots\).
    3. What are the corresponding points for the tent map?
  2. Find the endpoints of the \(\mathsf{LL}\), \(\mathsf{LR}\), \(\mathsf{RR}\), and \(\mathsf{RL}\) intervals for \(f\). Do the same for the \(\mathsf{LLL}\), \(\mathsf{LLR}\), \(\mathsf{LRR}\), \(\mathsf{LRL}\), \(\mathsf{RRL}\), \(\mathsf{RRR}\), \(\mathsf{RLR}\), and \(\mathsf{RLL}\) intervals.
    1. Find the itinerary of the point \(1.6\) under \(f\).
    2. Find the Lyapunov number of the periodic cycle you found in part (a)
    3. Which point in \([0,1]\) has the same itinerary under the tent map?
    1. The function \(f\) has two \(3\)-cycles. Find them. (Express your answers as fractions.)
    2. Determine the Lyapunov numbers of the cycles that you found in part (a).
    3. Find the two \(3\)-cycles for the tent map.
  5. To show that the dynamical systems determined by \(f\) and \(T\) are topologically conjugate, we must find a homeomorphism \(c\colon[0,1]\to[0,8]\) such that \(c\circ T = f\circ c\).
    1. Use questions 1–4 to list twenty-one data points for the function \(c\) (two from question 1, nine from question 2, four from question 3, and six from question 4).
    2. Find the formula for a function \(c\) whose graph includes all \(21\) data points. Verify this by plotting a graph of \(c\) together with the data points.
    3. Use Mathematica to verify that your formula for \(c\) satisfies \(c\circ T = f\circ c\).